Preasure Head Reduction

The Root Water Uptake Parameters for the water stress response function suggested by Feddes et al. [1978] are described in detail in the HYDRUS technical manual. Water uptake is assumed to be zero close to saturation (i.e. wetter than some arbitrary "anaerobiosis point" P0). Root water uptake is also zero for (negative) pressure heads less than the wilting point (P3). Water uptake is considered optimal between pressure heads Popt and P2, whereas for pressure heads between P2 and P3 (or P0 and Popt) water uptake decreases (or increases) linearly with pressure head.

The Root Water Uptake Parameters for the water stress response function suggested by Feddes et al. [1978] (above) are described in detail in the HYDRUS technical manual. Water uptake in this model is assumed to be zero close to saturation (i.e. wetter than some arbitrary “anaerobiosis point” P0). Root water uptake is also zero for pressure heads less (more negative) than the wilting point (P3). Water uptake is considered optimal between pressure heads Popt and P2, whereas for pressure heads between P2 and P3 (or P0 and Popt) water uptake decreases (or increases) linearly with pressure head.

P0	Value of the pressure head [L] below which roots start to extract water from the soil.
POpt	Value of the pressure head [L] below which roots extract water at the maximum possible rate.
P2H	Value of the limiting pressure head [L] below which roots cannot longer extract water at the maximum rate (assuming a potential transpiration rate of r2H).
P2L	As P2H, but for a potential transpiration rate of r2L.
P3	Value of the pressure head [L] below which root water uptake ceases (usually taken at the wilting point).
r2H	Potential transpiration rate [LT-1] (currently set at 0.5 cm/day).
r2L	Potential transpiration rate [LT-1] (currently set at 0.1 cm/day).

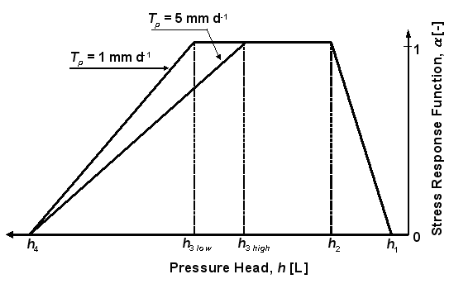
The above input parameters permit one to make the variable P2 a function of the potential transpiration rate, Tp (P2 presumably decreases at higher transpiration rates). HYDRUS currently implements the same linear interpolation scheme as used in several versions of the SWATRE code (e.g., Wesseling and Brandyk, 1985). The interpolation scheme is defined in the manual.

A database of suggested parameter values for the Feddes et al. [1978] model for different plants is provided based on studies by Wesseling [1991] and Taylor and Ashcroft [1972].

Potential transpiration rates [LT-1] r2L and r2H:

Potential transpiration rates [LT-1] r2L and r2H (currently set at 0.1 cm/day and 0.5 cm/day) permit one to make the variable h3 a function of the potential transpiration rate, Tp (h3 presumably decreases at higher transpiration rates). HYDRUS currently implements the same linear interpolation scheme as used in several versions of the SWATRE (e.g., Wesseling and Brandyk [1985]) and SWMS_2D [Šimůnek et al., 1992] codes. The scheme is based on the following interpolation:

P2=P2H+(P2L-P2H)/(r2H-r2L)*(r2H-Tp) for r2L<Tp<r2H

P2=P2L for Tp<r2L

P2=P2H for Tp>r2H

You can specify as many potential evaporation rates as you wish in the "Time-Variable Boundary Conditions" dialog windows. The values r2L and r2H do not have anything to do with that. As long as the soil is wet and the pressure head is larger then h3, the actual uptake will be the same as potential uptake. Only when the soil dries out to less that h3 will the potential uptake be reduced to the actual uptake. The point in time when this reduction starts then depends on the values of r2L and r2H. Some believe that this initial reduction (r2L) should depend on the potential transpiration rate (i.e., if the potential transpiration is larger the reduction starts earlier). The initial reduction becomes independent of potential transpiration if one specifies that r2L = r2H and P2L= P2L