Table of Anisotrophy Tensors

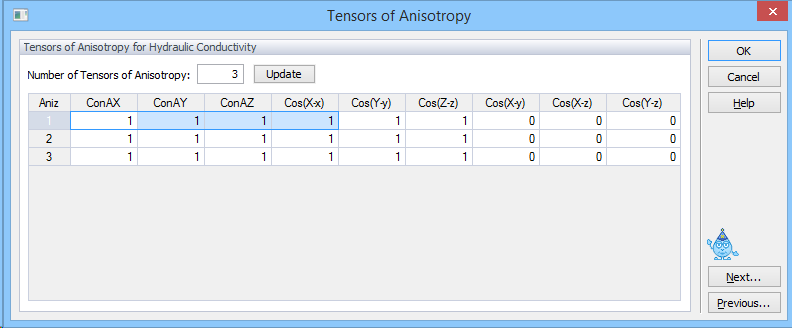
Definition of anisotropy has been simplified for three-dimensional problems where user can specify one or more Tensors of Anisotropy, which may be assigned to different parts of the transport domain. The anisotropy tensor is defined by three principal components, K1A (ConAX), K2A (ConAY), and K3A (ConAZ), and six coefficients aij that represent the cosine of angles between the ith principal direction of the tensor KA and the j-axis of the global coordinate system (i.e., Cos(X-x), Cos(Y-y), Cos(Z-y), Cos(X-y), Cos(X-z), Cos(Y-z)).

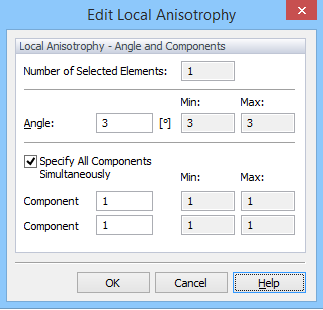
For two-dimensional problems users may need to specify the principal components of the anisotropy tensor, K1A and K2A, together with the angle ωa between the principal direction of K1A and the x‑axis of the global coordinate system for each element.